The time value of money is a basic financial concept that holds that money in the present is worth more than the same sum of money to be received in the future. This is true because money that you have right now can be invested and earn a return, thus creating a larger amount of money in the future.
(Also, with future money, there is the additional risk that the money may never actually be received, for one reason or another). The time value of money is sometimes referred to as the net present value (NPV) of money.
- How does the Time Value of Money Work?
- What is Time Value of Money With Example?
- Why do we Calculate Time Value of Money?
- What are the Three Techniques of TVM?
- How do you Calculate the Amount of Money?
- Time Value of Money Formula
- What are the Components of TVM?
- How do you Apply the time value of money?
- Which Technique is Most Appropriate Based on Time Value of Money?
How does the Time Value of Money Work?
A simple example can be used to show the time value of money. Assume that someone offers to pay you one of two ways for some work you are doing for them: They will either pay you $1,000 now or $1,100 one year from now.
Read Also: Can you Make Money From Coding?
Which pay option should you take? It depends on what kind of investment return you can earn on the money at the present time. Since $1,100 is 110% of $1,000, then if you believe you can make more than a 10% return on the money by investing it over the next year, you should opt to take the $1,000 now.
On the other hand, if you don’t think you could earn more than 9% in the next year by investing the money, then you should take the future payment of $1,100 – as long as you trust the person to pay you then.
What is Time Value of Money With Example?
The time value of money (TVM) is the concept that a sum of money is worth more now than the same sum will be at a future date due to its earnings potential in the interim. The time value of money is a core principle of finance. A sum of money in the hand has greater value than the same sum to be paid in the future. The time value of money is also referred to as the present discounted value.
Investors prefer to receive money today rather than the same amount of money in the future because a sum of money, once invested, grows over time. For example, money deposited into a savings account earns interest. Over time, the interest is added to the principal, earning more interest. That’s the power of compounding interest.
If it is not invested, the value of the money erodes over time. If you hide $1,000 in a mattress for three years, you will lose the additional money it could have earned over that time if invested. It will have even less buying power when you retrieve it because inflation reduces its value.
As another example, say you have the option of receiving $10,000 now or $10,000 two years from now. Despite the equal face value, $10,000 today has more value and utility than it will two years from now due to the opportunity costs associated with the delay. In other words, a delayed payment is a missed opportunity.
The time value of money has a negative relationship with inflation. Remember that inflation is an increase in the prices of goods and services. As such, the value of a single dollar goes down when prices rise, which means you can’t purchase as much as you were able to in the past.
Why do we Calculate Time Value of Money?
The Time Value of Money concept determines the potential earning capacity of an amount in the future. It, therefore, helps different financial sectors to understand and compute the present value and compare the same with the future value of a particular amount. Based on the results obtained, they decide whether to invest in a particular venture, asset, or security.
The financial firms use this idea of TVM for the following purposes:
- It helps in comparing the investment alternatives available in the market. Investors assess the returns and other conditions to make a final decision on what option to choose.
- Investors choose the best investment proposals based on the evaluation, considering the TVM.
- Lenders decide the interest rates for loans, mortgages, etc., based on the present and future value of an amount.
- The value of money, when known, helps in fixing appropriate wages and prices of products.
In addition, the changing value of an amount also plays a considerable role in determining when a particular investment matures or when to repay a loan amount, etc.
The three main reasons that make TVM an important concept are – inflation, risk or uncertainty, and liquidity.
• Inflation is the loss of purchasing power caused by the deteriorating future value of money.
• Risk or uncertainty is the difference between what is received as an outcome and expected when the investment or expenditure was made.
• Liquidity makes it easy for owners to sell their assets for cash as illiquid assets are difficult to sell.
What are the Three Techniques of TVM?
If you could choose between getting $500 now or getting $500 a year from now, most people would take the money now. This fundamental axiom involves the time value of money, and economists have sought ways to compare streams of money earned at different times in a way that takes time value into account.
Below, you’ll learn about three techniques that you can use in various situations to get the answers you’re looking for.
Present value calculations
One common time-value problem deals with expecting a specified sum of money at a point in the future. Because money earned in the future is worth less than money earned now, you have to apply a discount to the future payment in order to get its equivalent present value.
Often, the discount rate used is equal to the prevailing risk-free rate for assets like Treasury securities without default risk. The further into the future the payment is, the greater the discount.
The math behind a present value calculation is a bit complicated but can be done with a basic calculator. To come up with present value, take 1 and add it to the discount rate used. Then raise that number to the power of the number of years in the future that you’ll receive the payment. Save the resulting figure, and then divide the future payment amount by that figure. The final result will be the present value.
For instance, say you know that you’ll receive $110.25 in two years and decide that a discount rate of 5% is appropriate. In that case, 1 plus 5% equals 1.05, and 1.05 raised to the second power is 1.1025. Divide $110.25 by 1.1025, and you get $100, which is the present value.
Future value calculations
Future value calculations work in the opposite manner. You’ll follow the same steps as you did for present value, adding 1 to the discount rate and then raising that number to the power of the number of years in the future that you’re measuring the future value. But then, you’ll need to multiply the result by the value of the current payment. The final result is the future value.
For instance, if you want to know the future value of $100 in two years assuming a rate of 5%, then 1 + 5% is 1.05, 1.05 raised to the second power is 1.1025, and $100 multiplied by 1.1025 is $110.25. As you can see, this matches up with the present value calculation above.
Recurring value techniques
The two methods discussed above work well for one-time payments, but other methods are better for recurring payments. You can always just calculate present or future value for each payment separately, but there are sometimes shortcuts available for common situations.
For instance, say you have an asset that pays a perpetual stream of income. You can’t calculate each payment separately, but the equation for its present value turns out to be quite simple: take the amount of each regular payment and divide it by the discount rate. So if you receive $100 each year and use a discount rate of 5%, then its present value is $100 / 5% = $2,000.
Knowing how to deal with time-value problems can save a lot of time and make it easier to compare streams of future payments. That way, you can make smarter decisions about money.
How do you Calculate the Amount of Money?
The Money Calculator finds the total amount of money by adding up the sum of each denomination of bills and coins. Dollar bills are traditionally called “banknotes” or “notes” so we use these terms in our money calculator.
Use this money calculator to add up the total amount of money for common denominations of bills, coins and rolled or bagged coins in these currencies:
- United States Dollar USD
- European Euro EUR
- Japanese Yen JPY
- British Pound Sterling GBP
- Australian Dollar AUD
- Canadian Dollar CAD
- Swiss Franc CHF
- Swedish Krona SEK
- Mexican Peso MXN
- New Zealand Dollar NZD
- Indian Rupee INR
- Philippine Peso PHP
Please contact me if you would like to use the calculator for an additional currency or rolled coins not listed here.
How to Calculate Total Amount of Money
To add up the total amount of cash and coins you have, first sort each note and coin according to value. Make a separate pile for each denomination and then count how many of each bill or coin value you have.
For each bill and coin value, multiply the number you have by the face value. For example, if you have 4 of $10 note multiply 4 × 10 to get $40. If you have 3 of the $5 note multiply 3 × 5 to get $15.
Add all of the totals together to calculate the total sum of money.
Steps to Calculate Money
- Sort cash and coins so that each denomination is in its own stack
- Make a separate count of how many bills or coins are in each stack
- For each stack, multiply the face value by the number of bills or coins in that stack
- Add the totals of all stacks together. This is the sum total of the money.
Time Value of Money Formula
The time value of money is an important concept not just for individuals, but also for making business decisions. Companies consider the time value of money in making decisions about investing in new product development, acquiring new business equipment or facilities, and establishing credit terms for the sale of their products or services.
A specific formula can be used for calculating the future value of money so that it can be compared to the present value:

Where:
FV = the future value of money
PV = the present value
i = the interest rate or other return that can be earned on the money
t = the number of years to take into consideration
n = the number of compounding periods of interest per year
Using the formula above, let’s look at an example where you have $5,000 and can expect to earn 5% interest on that sum each year for the next two years. Assuming the interest is only compounded annually, the future value of your $5,000 today can be calculated as follows:
FV = $5,000 x (1 + (5% / 1) ^ (1 x 2) = $5,512.50
Present Value of Future Money Formula
The formula can also be used to calculate the present value of money to be received in the future. You simply divide the future value rather than multiply the present value. This can be helpful in considering two varying present and future amounts.
In our original example, we considered the options of someone paying your $1,000 today versus $1,100 a year from now. If you could earn 5% on investing the money now, and wanted to know what present value would equal the future value of $1,100 – or how much money you would need in hand now in order to have $1,100 a year from now – the formula would be as follows:
PV = $1,100 / (1 + (5% / 1) ^ (1 x 1) = $1,047
The calculation above shows you that, with an available return of 5% annually, you would need to receive $1,047 in the present to equal the future value of $1,100 to be received a year from now.
To make things easy for you, there are a number of online calculators to figure the future value or present value of money.
Net Present Value Example
Below is an illustration of what the Net Present Value of a series of cash flows looks like. As you can see, the Future Value of cash flows are listed across the top of the diagram and the Present Value of cash flows are shown in blue bars along the bottom of the diagram.

What are the Components of TVM?
The key components are as mentioned below –
1. Interest/Discount Rate (i)– It’s the rate of discounting or compounding that we apply to an amount of money to calculate its present or future value.
2. Time Periods (n) – It refers to the whole number of time periods for which we want to calculate the present or future value of a sum. These time periods can be annually, semi-annually, quarterly, monthly, weekly, etc.
3. Present value (PV)– The amount of money that we obtain by applying a discounting rate on the future value of any cash flow.
4. Future value (FV)– The amount of money that we obtain by applying a compounding rate on the present value of any cash flow.
5. Installments (PMT)– Installments represent payments to be paid periodically or received during each period. The value is positive when payments have been received and become negative when payments are made.
How do you Apply the time value of money?
The concept of the time value of money will be relevant in any situation where a party will be receiving or paying a sum of money to a third party.
That being said, let us discuss two everyday situations where we might have to apply the concepts of the time value of money.
Loan EMIs
EMIs, short for Equated Monthly Installments, constitute the prime method of repaying back a loan.
The need for the concept of time value arises when it comes to calculating the value of the EMIs. Let us return to the example we had talked about before.
As per the example, you went to purchase a bike worth Rs. 3,00,000 and the dealer gives you a choice.
- You either pay Rs 3,00,000 now, or
- Opt for installments of Rs 1,00,000 at the end of each year for the next three years.
Let’s evaluate each choice one by one.
The first one is pretty simple, right? You can just pay off Rs. 3,00,000 this instant, but this amount can cause a huge dent in your savings, depending on your income and financial status.
Now, for the second one, if you pay Rs. 1,00,000 at the end of each year for three years, it might cause you a bigger dent in your savings because as we’ve learned, the value of money keeps increasing over time.
Therefore, it is clear that even if we are paying three installments of Rs. 1,00,000 each, the effective value of these installments can be much higher in the future.
It’s because the installments are not the present values, whereas the Rs. 3,00,000 is the present value of your purchase.
One should always make sure that they are either comparing two future values or two current values to come to a correct investment decision.
Therefore, to make the correct decision, you should evaluate the present value of all the installments, add up their value, and then compare that value to the current price of the product to see how much interest you’re paying on the product.
This technique is relevant for any loan – from a home loan to a simple EMI-system purchase of home appliances.
Value of Investments in Bonds
The PV formula plays a pivotal role when it comes to calculating the yield to maturity (YTM) of a bond.
What is the Yield to Maturity?
In simple terms, the YTM is the rate of return that shows the total anticipated return to an investor if they hold the bond till maturity.
Consider this – suppose an investor wants to invest in a security that will yield them an interest rate of at least 10%.
How will the investor be able to decide if the bonds they want to purchase will give them as many returns?
Simple! Calculating the YTM requires the calculation of the PV of every cash flow from the bond to the investor.
Therefore, the investor can compare the YTM rate with their desired rate of interest to decide if the bonds are a suitable purchase for them.
In this way, the concept of time value is essential for an investor looking to buy bonds in the future.
Which Technique is Most Appropriate Based on Time Value of Money?
There are two techniques of estimating time value of money which are discussed below
Technique # 1. Discounting or Present Value Method:
The current value of an expected amount of money to be received at a future date is known as Present Value. If we expect a certain sum of money after some years at a specific interest rate, then by discounting the Future Value we can calculate the amount to be invested today, i.e., the current or Present Value.
Read Also: What is Financial Planning Process?
Hence, Discounting Technique is the method that converts Future Value into Present Value. The amount calculated by Discounting Technique is the Present Value and the rate of interest is the discount rate. Discounting can be done a number of times and based on this, methods for calculating Present Values are listed as follows in Table 2.2 –

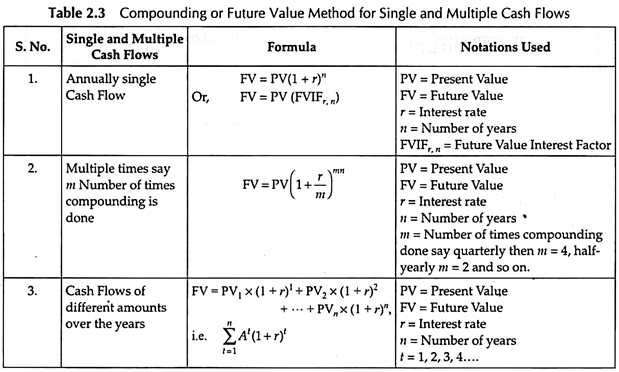
Technique # 2. Compounding or Future Value Method:
Compounding is just the opposite of discounting. The process of converting Present Value into Future Value is known as compounding.
Future Value of a sum of money is the expected value of that sum of money invested after n number of years at a specific compound rate of interest.
Methods for calculating Future Value are given as follows Table 2.3 –